A recent book by Alexander Unzicker “The Higgs Fake” considers, in particular, how “particle physicists are fooling themselves with alleged results, while their convictions are based on group-think and parroting.” It represents a critical point of view and it is not groundless. I would like to support Alexander Unzicker in his critics. In former times the founding fathers of physics were speaking of non resolved fundamental problems, which are still not resolved satisfactorily, but nowadays everything is represented as a fulfledged building based on some “fundamental principles”. Let us take, for example, a citation of W. Pauli, one of most honest physicist of the last century:
“We will be considered the generation that left behind unsolved such essential problems as the electron self-energy.“
I think the essence is here and avoiding it created just a shaman’s practice where cheating and self-fooling are essential parts. To prove that, let me be more specific and let us consider the electron electromagnetic mass. This notion had arisen in Classical Electrodynamics (CED) well before the famous  was derived [1] and it remains an unsolved problem even today (there are still publications on this subject). We must not confuse it with the electromagnetic mass defect, though, i.e., with a calculable interaction energy.
was derived [1] and it remains an unsolved problem even today (there are still publications on this subject). We must not confuse it with the electromagnetic mass defect, though, i.e., with a calculable interaction energy.
The electromagnetic mass can be thought of as a Coulomb energy of the electric field surrounding the electron when we calculate the total field energy. In other words, it is a consequence of the field concept. This part of the field energy is cut-off dependent and thus can take any value at your convenience. We all are familiar with the classical radius of electron 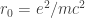 , but if we take into account the electron magnetic moment field energy too, we will obtain another radius, closer to the Compton length
, but if we take into account the electron magnetic moment field energy too, we will obtain another radius, closer to the Compton length 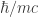 . Still, in nature there is no electron of the classical or any other radius. And normally this part of the field energy is entirely discarded and what is left is an interaction energy of charges. Thus, when we calculate a field energy, the electromagnetic mass is just of no use.
. Still, in nature there is no electron of the classical or any other radius. And normally this part of the field energy is entirely discarded and what is left is an interaction energy of charges. Thus, when we calculate a field energy, the electromagnetic mass is just of no use.
Apart from the total field energy, the electromagnetic mass of a point-like charge  enters the usual “mechanical” equation of a charge when we decide to insert the charge proper field into the charge equation of motion in the frame of a self-action ansatz. The latter is done for the sake of taking into account a weak radiation reaction force, which must provide the total energy conservation. The motivation – energy conservation – is understandable, but in a field approach with
enters the usual “mechanical” equation of a charge when we decide to insert the charge proper field into the charge equation of motion in the frame of a self-action ansatz. The latter is done for the sake of taking into account a weak radiation reaction force, which must provide the total energy conservation. The motivation – energy conservation – is understandable, but in a field approach with 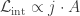 , we insert the entire filed
, we insert the entire filed  , not just a radiation field
, not just a radiation field  , in the mechanical equation. We do it by (a wrong) analogy with an external force
, in the mechanical equation. We do it by (a wrong) analogy with an external force 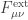 . So, before this our intervention we have a good “mechanical” equation (I use a non relativistic form)
. So, before this our intervention we have a good “mechanical” equation (I use a non relativistic form)

which works almost fine (the near field, whatever it is, easily follows the charge according to Maxwell equations), and after our noble intervention it becomes
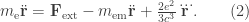
that does not work any more. The corresponding self-force term  with
with 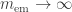 makes it impossible for a charge to change its state of a uniform motion
makes it impossible for a charge to change its state of a uniform motion  . This is a self-induction force, an extremely strong one. It’s an understandable “physical effect”, but first, it is not observed as infinite, and second, the self-induction force is not a radiation reaction force in any way, so our approach to describing the radiation influence via self-action is blatantly wrong. Albeit of an anticipated sign (and even when made finite and small), it does not help conserve the total energy. Microsoft Windows would say:
. This is a self-induction force, an extremely strong one. It’s an understandable “physical effect”, but first, it is not observed as infinite, and second, the self-induction force is not a radiation reaction force in any way, so our approach to describing the radiation influence via self-action is blatantly wrong. Albeit of an anticipated sign (and even when made finite and small), it does not help conserve the total energy. Microsoft Windows would say:

I. e., the term  is not of the right functional dependence. Instead of recognizing this error, physicists started to search a pretext to keep to the self-action idea in place. They noticed that discarding the term
is not of the right functional dependence. Instead of recognizing this error, physicists started to search a pretext to keep to the self-action idea in place. They noticed that discarding the term  “helps” (we will later see how it helps), but calling it honestly “discarding” makes fun of physicists. Discarding is not a calculation. Thus, another brilliant idea was advanced – an idea of “bare” mass
“helps” (we will later see how it helps), but calling it honestly “discarding” makes fun of physicists. Discarding is not a calculation. Thus, another brilliant idea was advanced – an idea of “bare” mass  that “absorbs”
that “absorbs”  (a “mechanism” called later a mass renormalization). Tricky is Nature, but clever are some physicists. In a fresh historical paper Kerson Huang expresses the common attitude to it [2]:
(a “mechanism” called later a mass renormalization). Tricky is Nature, but clever are some physicists. In a fresh historical paper Kerson Huang expresses the common attitude to it [2]:
“One notices with great relief that the self‐mass can be absorbed into the physical mass in the equation of motion“
and he writes down an equation, which experimentally follows from nowhere:
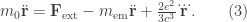
It is namely here where the negative bare mass  is introduced in physics by physicists, introduced exclusively with the purpose to subtract the harmful electromagnetic mass. This introduction is not convincing to me. A negative mass makes the particle move to the left when the force pulls it to the right. We never observed such a silly behaviour (like that of a stupid goat) and we never wrote the corresponding equations. We cannot pretend that (1) describes such a wrong particle in an external field, but adding its self-induction makes the equation right, as it does Kerson Huang. It’s all the way around: in order to make the wrong equation (2) closer to the original one (1), we just discard the electromagnetic mass whatever value it takes. Kerson Huang should have written honestly “One notices that the self‐mass ought to be omitted“.
is introduced in physics by physicists, introduced exclusively with the purpose to subtract the harmful electromagnetic mass. This introduction is not convincing to me. A negative mass makes the particle move to the left when the force pulls it to the right. We never observed such a silly behaviour (like that of a stupid goat) and we never wrote the corresponding equations. We cannot pretend that (1) describes such a wrong particle in an external field, but adding its self-induction makes the equation right, as it does Kerson Huang. It’s all the way around: in order to make the wrong equation (2) closer to the original one (1), we just discard the electromagnetic mass whatever value it takes. Kerson Huang should have written honestly “One notices that the self‐mass ought to be omitted“.
As well, those who refer to a hydrodynamics analogy, present this silly speculation about arbitrary  and
and  as a typical calculation, a calculation like in hydrodynamics where everything is separately measurable, known, and physical. In CED it is not the case. And if the electromagnetic mass is present already in our phenomenological equation (1), the method of self-action takes it into account once more which shows again that such an approach is self-inconsistent. You know, self-induction of a wire is in fact a completely calculable physical phenomenon occurring with many interacting charges. Similarly in plasma description we calculate interactions for dynamics. Interaction is a good concept, but a self-action of an elementary particle is a bad idea. It describes no internal dynamics by definition.
as a typical calculation, a calculation like in hydrodynamics where everything is separately measurable, known, and physical. In CED it is not the case. And if the electromagnetic mass is present already in our phenomenological equation (1), the method of self-action takes it into account once more which shows again that such an approach is self-inconsistent. You know, self-induction of a wire is in fact a completely calculable physical phenomenon occurring with many interacting charges. Similarly in plasma description we calculate interactions for dynamics. Interaction is a good concept, but a self-action of an elementary particle is a bad idea. It describes no internal dynamics by definition.
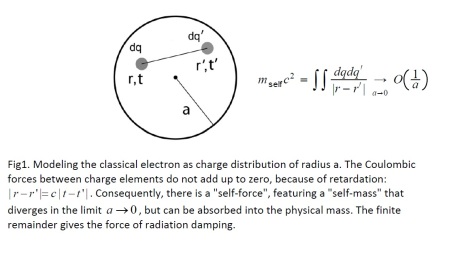
If a bare particle is truly not observable, we cannot even establish an equation for it and we cannot pretend that its equation is of the same form as the Newton equations for physical particles. That is why they say that the bare mass is not observable alone – it always comes in (3) together with the electromagnetic one: 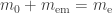 . But it is not true either: equation (1) contains the physical mass
. But it is not true either: equation (1) contains the physical mass 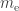 and in addition, if the external force in (1) contains the omnipresent gravity force, say,
and in addition, if the external force in (1) contains the omnipresent gravity force, say, 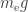 for simplicity, the latter does not acquire any addendum when we add that self-induction force. In reality, we fight our own invention
for simplicity, the latter does not acquire any addendum when we add that self-induction force. In reality, we fight our own invention  with help of another one –
with help of another one –  , but too many people believe in both.
, but too many people believe in both.
This is the real truth about mass “renormalization” procedure. We ourselves introduce the self-mass in our equation and then we remove it. As nothing remains from it anywhere (the physical mass stays intact), I can safely say that there is no electromagnetic mass at all, that’s my answer to this question (again, not to confuse with the mass defect due to interaction). (By the way, renormalization does not work without fail – there are many non renormalizable theories where bad interaction terms spoil not only the original equation coefficients, but also introduce wrong “remainders”. Success of renormalization is based on lucky accidents, see my opus here or here. P. Dirac clearly called it a fluke.)
Those who insist on this “calculation” forget that then there are forces keeping the charge parts together and these forces have their own “self-induction” and “radiation reaction” contributions. No, this model needs too many “unknowns”.
Here I naively wonder why not from the very beginning to use just the radiated field instead of the total field to take into account the “radiation reaction”? Then they might never obtain the harmful jerk term  , but they do not do it. They stick to the self-action patched with the “bare mass mechanism” and they hope that the jerk “remainder” of self-action will correctly describe the radiation reaction. Let us see.
, but they do not do it. They stick to the self-action patched with the “bare mass mechanism” and they hope that the jerk “remainder” of self-action will correctly describe the radiation reaction. Let us see.
So, after shamefully camouflaging discarding silly  , they are left with the jerk
, they are left with the jerk 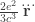 called a “radiation reaction” force:
called a “radiation reaction” force:
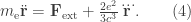
Fortunately, it is wrong too. I say “fortunately” because it reinforces my previous statement that the self-action is a wrong idea. This remainder cannot be used as it gives runaway solutions. Not a small radiation reaction, but a rapid self-acceleration. Microsoft Windows would say:

I. e., the term 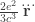 is not of the right functional dependence either. In other words, all terms of self-action force in (2) are wrong. Some textbook authors are even happy that the jerk term does not depend on the charge shape, but I personally think such a force is not physical either – precisely because of this “lucky” feature. Briefly, this self-action idea was tried and it failed miserably. Period.
is not of the right functional dependence either. In other words, all terms of self-action force in (2) are wrong. Some textbook authors are even happy that the jerk term does not depend on the charge shape, but I personally think such a force is not physical either – precisely because of this “lucky” feature. Briefly, this self-action idea was tried and it failed miserably. Period.
(This self-action can be figuratively represented as connecting an amplifier output to its input. It creates a feedback. First the feedback is strongly negative – no reaction to an external signal is possible anymore. After “repairing” this undesirable feedback, we get a strong positive feedback. Now we have a self-amplification whatever the external signal value is. No good either.)
A. Unzicker speaks of a fake and for some readers this may look as an exaggeration. If you want to see physicists cheating, here is another bright example. This cheating consists in using 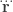 in their “proof” of energy conservation [3], as if the corresponding equation (4) had physically reasonable quasi-periodical solutions. But it doesn’t! Runaway solutions are not quasi-periodical and are not physical at all, so the proof is just a deception. (They multiply
in their “proof” of energy conservation [3], as if the corresponding equation (4) had physically reasonable quasi-periodical solutions. But it doesn’t! Runaway solutions are not quasi-periodical and are not physical at all, so the proof is just a deception. (They multiply 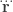 by
by  and integrate it by parts to “show” that on average it is a radiation power.) If they insist on using quasi-periodic solutions in their proof, these solutions do not belong to Eq. (4). (A “jerky” equation like (4) does not even have any physical Lagrangian to be directly derived from!)
and integrate it by parts to “show” that on average it is a radiation power.) If they insist on using quasi-periodic solutions in their proof, these solutions do not belong to Eq. (4). (A “jerky” equation like (4) does not even have any physical Lagrangian to be directly derived from!)
As a matter of fact, after cheating with the “proof“, this harmful jerk term is also (quietly) abandoned in favor of some small force term used in practice instead. This small term is  (or alike):
(or alike):

Equation (5) is much better, but here again, I notice cheating once more because they represent it as a “derivation” from (4). Now cheating consists in replacing 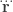 with
with  as if we solved (4) by iterations (perturbation method). However, in the true iterative procedure we obtain a given function of time
as if we solved (4) by iterations (perturbation method). However, in the true iterative procedure we obtain a given function of time 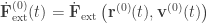 on the right-hand side rather than a term
on the right-hand side rather than a term  expressed via unknown dynamical variables
expressed via unknown dynamical variables  and
and 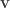 . For example, in an oscillator equation
. For example, in an oscillator equation
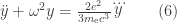
the first perturbative term 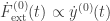 is a known external (periodic and resonance (!) in case of harmonic oscillator) driving force whereas the replacement term
is a known external (periodic and resonance (!) in case of harmonic oscillator) driving force whereas the replacement term 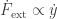 is unknown damping force (kind of a friction):
is unknown damping force (kind of a friction):

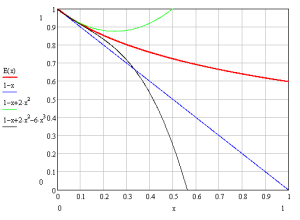
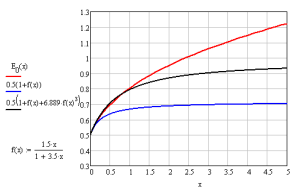
A perturbative solution to (6) 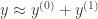 (a red line in Fig. 2)
(a red line in Fig. 2)
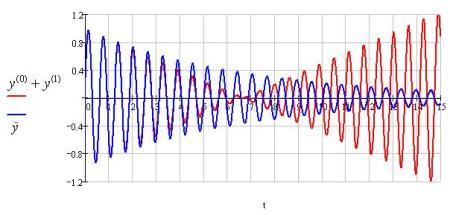 Fig. 2.
Fig. 2.
is different from a damped oscillator solution  (a blue line in Fig. 2). Solution to a damped oscillator equation is non linear in
(a blue line in Fig. 2). Solution to a damped oscillator equation is non linear in 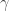 , non linear in a quite certain manner. It is not a self-action, but an interaction with something else. This difference in equations is qualitative (conceptual) and it is quantitatively important in case of a strong radiation reaction force and/or when
, non linear in a quite certain manner. It is not a self-action, but an interaction with something else. This difference in equations is qualitative (conceptual) and it is quantitatively important in case of a strong radiation reaction force and/or when  (I used in this example
(I used in this example  with
with  , and
, and 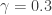 ). I conclude therefore that a damped oscillator equation (7) is not a perturbative version of (6), but is another guesswork result tried and left finally in practice because of its physically more reasonable (although still approximate) behaviour. Similarly, equation (5) is not a perturbative version of (4), but another (imperceptible) equation replacement [3], [4]. Of course, there is no and may not be any proof that perturbative series for (4) converge to solutions of (5). The term
). I conclude therefore that a damped oscillator equation (7) is not a perturbative version of (6), but is another guesswork result tried and left finally in practice because of its physically more reasonable (although still approximate) behaviour. Similarly, equation (5) is not a perturbative version of (4), but another (imperceptible) equation replacement [3], [4]. Of course, there is no and may not be any proof that perturbative series for (4) converge to solutions of (5). The term  is a third functional dependence tried for description of the radiation reaction force.
is a third functional dependence tried for description of the radiation reaction force.
Hence, researchers have been trying to derive equations describing the radiation reaction force correctly, but they’ve failed. For practical (engineering) purposes they constructed (found by trying different functions) and are content with approximate equations like (5) that do not provide the exact energy conservation and do not follow from “principles” (no Lagrangian, no Noether theorem, etc.). Factually the field approach has been “repaired” several times with anti-field guesswork, if you like. Anyway, we may not represent it as a continuous implementation of principles because it isn’t so.
Guessing equations, of course, is not forbidden, on the contrary, but this story shows how far away we have gone from the original idea of self-action. It would not be such a harmful route if the smart mainstream guys did not raise every step of this zigzag guesswork into “the guiding principles” – relativistic and gauge invariance, restricting, according to the mainstream opinion, the form of interaction to  . Nowadays too few researchers see these steps as a severe lack of basic understanding of what is going on. On the contrary, the mainstream ideology consists in dealing with the same wrong self-action mechanism patched with the same discarding prescription (“renormalization”), etc., but accompanied also with anthems to these “guiding principles” and to their inventors. I do not buy it. I understand the people’s desire to look smart – they grasped principles of Nature, but they look silly to me instead.
. Nowadays too few researchers see these steps as a severe lack of basic understanding of what is going on. On the contrary, the mainstream ideology consists in dealing with the same wrong self-action mechanism patched with the same discarding prescription (“renormalization”), etc., but accompanied also with anthems to these “guiding principles” and to their inventors. I do not buy it. I understand the people’s desire to look smart – they grasped principles of Nature, but they look silly to me instead.
Indeed, let us forget for a moment about its inexactness and look at Eq. (5) as at an exact equation, i.e., as containing the desirable radiation reaction correctly. We see, such an equation exists (at least, we admit its existence), it does not contain any non physical stuff like  and
and  , and together with Maxwell equations it works fine. Then why not to obtain (5) directly from (1) and from another physical concept different from a wrong self-action idea patched with several forced replacements of equations? Why do we present our silly way as the right and unique? Relativistic and gauge invariance (equation properties) must be preserved, nobody argues, but making them “guiding principles” only leads to catastrophes, so (5) it is not a triumph of “principles”, but a lucky result of our difficult guesswork done against the misguiding principles. Principles do no think for us researchers. Thinking is our duty. Factually we need in (1) a small force like that in (5), but our derivation gives (2). What we then do is a lumbering justification of replacements of automatically obtained bad functions with creatively constructed better ones. Although equations like (5) work satisfactorily in some range of forces, the lack of mechanical equation with exact radiation reaction force in CED shows that we have not reached our goal and those principles have let us down.
, and together with Maxwell equations it works fine. Then why not to obtain (5) directly from (1) and from another physical concept different from a wrong self-action idea patched with several forced replacements of equations? Why do we present our silly way as the right and unique? Relativistic and gauge invariance (equation properties) must be preserved, nobody argues, but making them “guiding principles” only leads to catastrophes, so (5) it is not a triumph of “principles”, but a lucky result of our difficult guesswork done against the misguiding principles. Principles do no think for us researchers. Thinking is our duty. Factually we need in (1) a small force like that in (5), but our derivation gives (2). What we then do is a lumbering justification of replacements of automatically obtained bad functions with creatively constructed better ones. Although equations like (5) work satisfactorily in some range of forces, the lack of mechanical equation with exact radiation reaction force in CED shows that we have not reached our goal and those principles have let us down.
Note, although the above is a non relativistic version of CED, the CED story is truly relativistic and gauge invariant and it serves as a model to many further theory developments. In particular, nowadays in QFT they “derive” the wrong self-action Lagrangian from a “principle of local gauge invariance” (a gauge principle for short). They find it beautiful mathematically, enjoy the equation symmetries and conservation laws that follow from this symmetry. They repeat QED where they think there is this “gauge principle”. However such gauge equations do not have physical solutions, so their conserved quantities are just a bullshit. During enjoying the beauty of gauge interaction, they omit to mention that the solutions are non physical. The gauge principle in QED does not lead to physical equations. We are forced to rebuild a gauge theory as I outlined above. In CED the bare and electromagnetic masses appear and disappear shortly after for good, but in QED and QFT they reappear in each perturbative order. In addition, the physical charge also acquires unnecessary and bad “corrections”, and their omnipresence makes an impression of their belonging to physics.
Next, new “principles” come into play – they come into play with the purpose to fix this shit. Those principles serve to “allow” multiple replacements of bad terms in solutions with better ones – bare stuff and renormalizations, of course. A whole “fairy science” about a “vacuum polarization” around a still “bare” charge is developed to get rid of bad perturbative corrections in this wrong gauge construction (renormalization group). It boils down to adding a counter-term Lagrangian 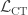 to the gauge one
to the gauge one  :
:
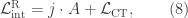
so the interaction becomes different from a purely gauge one. (Often it is presented as imposing physical conditions to a (bad) theory.) Thus, bare stuff and bad corrections cancel each other and do not exits any more. That’s their fate – to disappear from physics forever, if you understand it right. And it is we who make them disappear, not physical phenomena like vacuum polarization, etc. In other words, renormalization is not a calculation, but a specific modification of calculation results.
But this fix is not sufficient either. One needs to sum up soft diagrams too (to all orders) in order to obtain physically meaningful results because, alas, the electron does not correctly radiate otherwise and calculation fails! The latter fact shows eloquently that some part of “perturbation” (8) (let’s call it figuratively 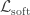 ) is not small and should be taken into account exactly (joined with
) is not small and should be taken into account exactly (joined with 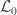 , hence, removed from the “perturbation”):
, hence, removed from the “perturbation”):
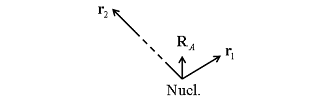
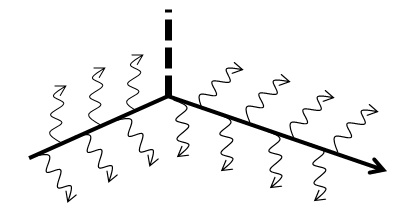 Fig. 3. Electron scattering from an external field in the first Born approximation, as it must be.
Fig. 3. Electron scattering from an external field in the first Born approximation, as it must be.

Such taking into account exactly is in fact using another, a more physical, zeroth-order approximation with Lagrangian 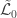 (9). The electron charge
(9). The electron charge  is involved there non perturbatively, so the electron is already coupled with the field variables, at least, partially (I call such an approximation an “electronium” [5]). Interaction (10) is even more different from the “gauge” one. (A good qualitative and quantitative analogy to such IR-divergent series and their exact sums is the second atomic form-factor
is involved there non perturbatively, so the electron is already coupled with the field variables, at least, partially (I call such an approximation an “electronium” [5]). Interaction (10) is even more different from the “gauge” one. (A good qualitative and quantitative analogy to such IR-divergent series and their exact sums is the second atomic form-factor 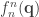 (3) and its series in powers of
(3) and its series in powers of  when
when  and
and  , see Fig. 3 in [5] and [7].)
, see Fig. 3 in [5] and [7].)
You see, our former initial approximation (decoupled electron in 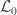 ) is not physical. You know why? Because we admit free particles in our minds and thus in equations. We observe interacting macroscopic bodies. In the simplest case we speak of a probe body in an external force. Sometimes the external forces add up into nearly zero and they do not change noticeably the body kinetic energy. Then we say the probe body is “free”. But we observe it with help of interactions too (inclusive image obtained with photons, for example), so it is never free, as a matter of fact, and, of course, its mass is not bare. For electron it also means that its very notion as a “point particle” and its equations is an inclusive picture of something compound [5]. An electron coupled within field oscillators has a natural mechanism of “radiation reaction” and a natural inclusive picture. Such a coupling is always on and never is off, unlike the gauge term
) is not physical. You know why? Because we admit free particles in our minds and thus in equations. We observe interacting macroscopic bodies. In the simplest case we speak of a probe body in an external force. Sometimes the external forces add up into nearly zero and they do not change noticeably the body kinetic energy. Then we say the probe body is “free”. But we observe it with help of interactions too (inclusive image obtained with photons, for example), so it is never free, as a matter of fact, and, of course, its mass is not bare. For electron it also means that its very notion as a “point particle” and its equations is an inclusive picture of something compound [5]. An electron coupled within field oscillators has a natural mechanism of “radiation reaction” and a natural inclusive picture. Such a coupling is always on and never is off, unlike the gauge term  treated perturbatively. W. Pauli always argued that one should look for a formulation of QED (or a field theory in general) which would mathematically not allow the description of a charged particle without its electromagnetic field. Now, seeing to what extent
treated perturbatively. W. Pauli always argued that one should look for a formulation of QED (or a field theory in general) which would mathematically not allow the description of a charged particle without its electromagnetic field. Now, seeing to what extent 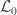 and
and  are different from (9) and (10), I can safely say that they really do not understand what to start with in their “gauge theories”. Even a physical solution of a partially coupled electron (a “hairy” electron line in Fig. 3) is not written, understood, and explained in QED, but who cares? (My mechanical [6] and atomic toy models [7] demonstrate that this can be achieved.)
are different from (9) and (10), I can safely say that they really do not understand what to start with in their “gauge theories”. Even a physical solution of a partially coupled electron (a “hairy” electron line in Fig. 3) is not written, understood, and explained in QED, but who cares? (My mechanical [6] and atomic toy models [7] demonstrate that this can be achieved.)
In electroweak unification they wanted to make the weak part of interaction to be a “gauge” too, but the gauge fields are massless. What a pity! Not only this construction needs counter-terms and soft diagram summations, now it needs a special “mechanism” to write down the mass terms in 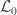 . Such a fix was found and it is known now as a Higgs mechanism. This fix to a bad gauge interaction idea is presented now as the ultimate explanation of the nature of mass: “Every ounce of mass found in the universe is made possible by the Higgs boson.” I wonder how were we doing before Higgs? With writing down phenomenological mass terms, we were in error, weren’t we? No. Then why all these complications? Because they do not know how to write down interactions with massive particles correctly (an old story, see (9) and (10) above). All they write is not only non physical, but also non renormalizable, so they decided to try here the gauge principle too. Fortunately or unfortunately, but some such constructions are renormalizable, thus they survived.
. Such a fix was found and it is known now as a Higgs mechanism. This fix to a bad gauge interaction idea is presented now as the ultimate explanation of the nature of mass: “Every ounce of mass found in the universe is made possible by the Higgs boson.” I wonder how were we doing before Higgs? With writing down phenomenological mass terms, we were in error, weren’t we? No. Then why all these complications? Because they do not know how to write down interactions with massive particles correctly (an old story, see (9) and (10) above). All they write is not only non physical, but also non renormalizable, so they decided to try here the gauge principle too. Fortunately or unfortunately, but some such constructions are renormalizable, thus they survived.
We remember the fiasco with the electron electromagnetic mass, and the Higgs proper mass is not really different since the Higgs boson acquires its own mass due to “self-action” too. It is not a calculation, but a fake since the Higgs boson mass is taken from experiment.
The Standard Model is also furnished with “fine tuning mechanism” because otherwise it is still a bullshit. And let me mention fitting parameters coming with the “Higgs mechanism”. Now the fitting properties of theory increased. Some, however, confuse it with increase of “predictive power”.
To me the Higgs is a fix, a fix somewhat similar to the bare mass term in CED compensating an obviously wrong construction, but a more complicated fix. I do not think it is an achievement. A bare mass notion is not an achievement in physics. The freedom in choosing the cutoff 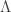 in a relationship
in a relationship  (à la renorm-group) is not physics,
(à la renorm-group) is not physics, 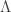 -independence of
-independence of 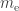 is not a CED “universality”. I hope I am clear here. But nowadays particle physics is stuffed with artefacts of our patches and stopgaps, so it is really difficult to distinguish what is physical and what is a fairy tale (a fake).
is not a CED “universality”. I hope I am clear here. But nowadays particle physics is stuffed with artefacts of our patches and stopgaps, so it is really difficult to distinguish what is physical and what is a fairy tale (a fake).
Today they sell you the bare stuff, its self-action dictated with the gauge principle, then counter-terms, IR diagram summation, Higgs field with self-action and fine tuning, poisons and antidotes, shit with nutlets, etc. as a physical theory. They are very pushy in that. They grasped all the principles of Nature.
No, they fool themselves with “clever insights” and fairy tales instead of doing physics. They count on “guiding principles”, they are under the spell of the gauge and other principles. Sticking to them is like being possessed. This fact underlines the shaky grounds the modern QFT is based on.
We have no right to dope ourselves with self-fooling and self-flattering. The conceptual problems have not been resolved, let us recognize it.
(To be updated.)
[1] Laurie M. Brown (editor). Renormalization From Lorentz to Landau (and beyond), 1993, Springer-Verlag, the talk of Max Dresden.
[2] Kerson Huang, A Critical History of Renormalization, http://arxiv.org/abs/1310.5533
[3] H. Lorentz, Landau-Lifshitz, R. Feynman, etc.
[4] Fritz Rohrlich, The dynamics of a charged particle, (2008) http://arxiv.org/abs/0804.4614
[5] Vladimir Kalitvianski, Atom as a “Dressed” Nucleus, Central European Journal of Physics, V. 7, N. 1, pp. 1-11 (2009), http://arxiv.org/abs/0806.2635
[6] Vladimir Kalitvianski, A toy model of renormalization and reformulation, http://arxiv.org/abs/1110.3702
[7] Vladimir Kalitvianski, On integrating out short-distance physics, http://arxiv.org/abs/1409.8326
(the remainder after the mass renormalization) is a good radiation reaction term [1, 2] even though it leads to “false start” solutions; others, on the contrary, teach that
is not applicable at “small times” and one must use
instead [3], but up to now no mechanical equation was found to conserve the energy-momentum exactly and in a physical manner. We content ourselves with an approximate description. The Lorentz covariance and the Noether theorem did not help [4], [5]!


 If you have read my blogs (this one, http://fishers-in-the-snow.blogspot.fr/ , http://vladimir-anski.livejournal.com/) and articles, (more here) you may have an idea what I mean by reformulation. If you like, my program can roughly be understood as both fulfilling the counter-term subtractions exactly:
If you have read my blogs (this one, http://fishers-in-the-snow.blogspot.fr/ , http://vladimir-anski.livejournal.com/) and articles, (more here) you may have an idea what I mean by reformulation. If you like, my program can roughly be understood as both fulfilling the counter-term subtractions exactly: will contain soft modes and physical constants by construction. Then the “interaction term” will be different too: